Relative Extrema

Relative Extrema#

Definitions#

Definition

A function f has a relative maximum at x=c if there exists an open interval (a,b) containing c such that f(c)≥f(x) for all x in (a,b). In this case, f(c) is called a relative maximum value of f.

Definition

A function f has a relative minimum at x=c if there exists an open interval (a,b) containing c such that f(c)≤f(x) for all x in (a,b). In this case, f(c) is called a relative minimum value of f.

Example 1#

Relative Extrema

The relative extrema are highlighted on the following graph. Observe how the relative extrema appear at points on the curve where the increasing/decreasing behavior of the function changes. In other words, relative extrema appear at points on the graph of the function where the derivative changes sign.

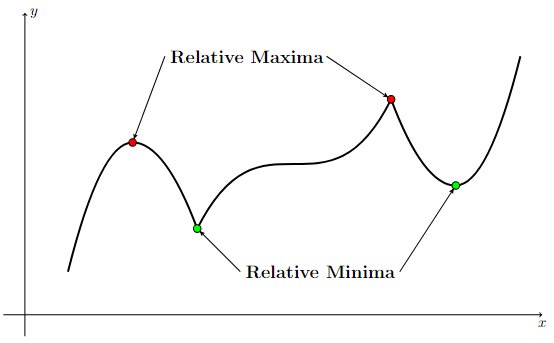
Graph of a generic function
Long Text Description

There is a horizontal x-axis. There is a vertical y-axis. The graph of a function is plotted on these axes. Moving from left to right, the function goes up towards a rounded corner, which is marked in red and labeled a relative maximum, then goes downward towards a sharp corner, which is marked in green and labeled a relative minimum, then goes up again towards another sharp corner, which is marked in red and labeled a relative maximum, then goes down again towards a rounded corner, which is marked in green and labeled a relative minimum, and then goes upward.

Observation About f′ Where Relative Extrema Appear

The relative extrema of a function appear where f′(x) changes from positive to negative or from negative to positive. Since f′(x) changes sign when there is a relative extrema, it must be the case that either f′(x)=0 or f′(x) does not exist at the relative extrema.